Hilbert Transform Envelope

Introduction

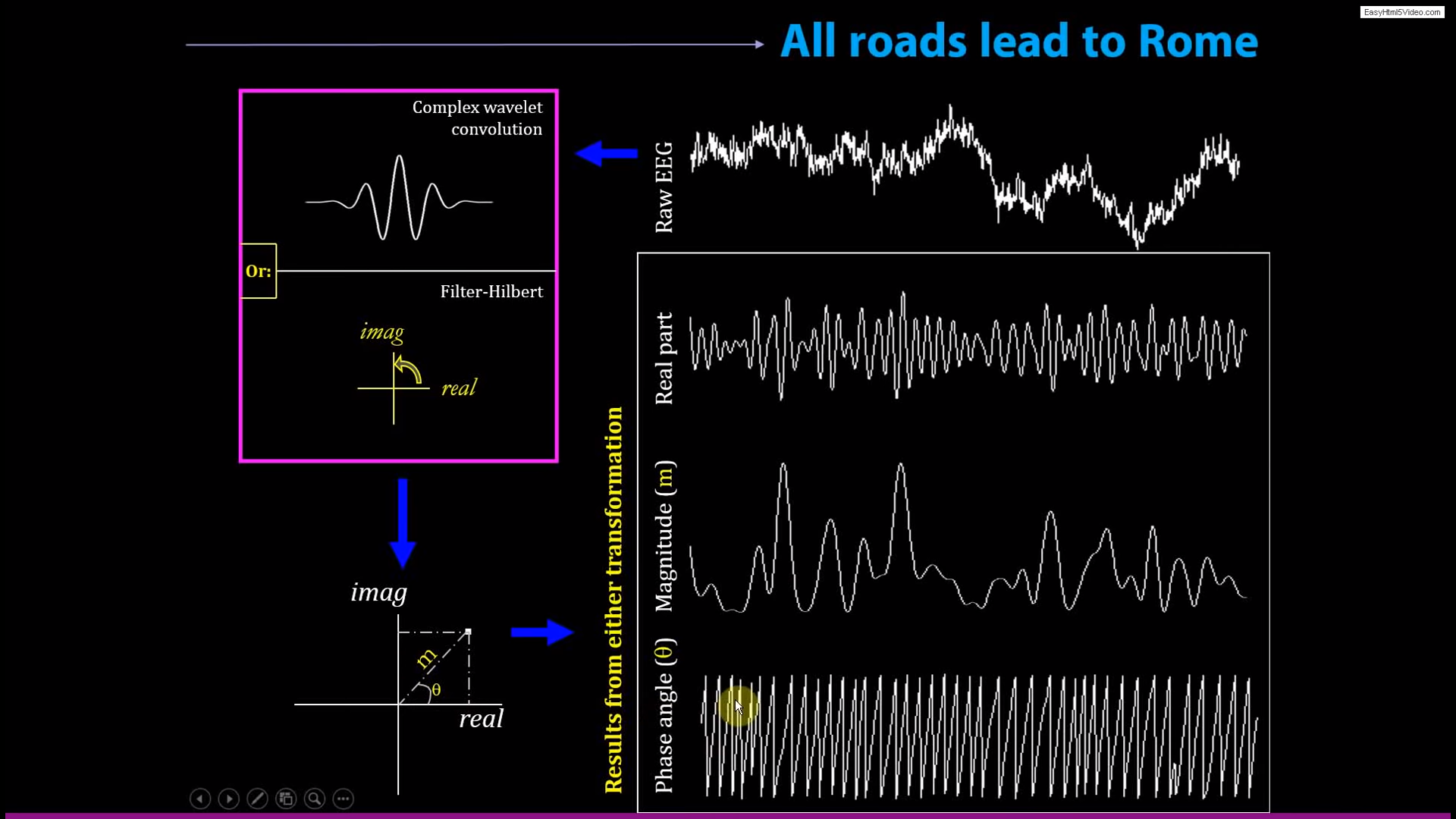
Envelope Explanation

Envelope and Fine Structure

• Envelope:
  • The envelope of a signal represents the slowly varying amplitude or outline of the signal. It provides a smooth curve that encapsulates the main shape of the signal, ignoring the rapid oscillations or fluctuations. The envelope is typically associated with the low-frequency components of a signal.
• Fine Structure:
  • The fine structure of a signal refers to the detailed, high-frequency components or rapid oscillations present in the signal. It captures the fast variations that occur on a shorter time scale compared to the envelope.

Algorithm Detail

History

• 1905年---Hilbert在研究黎曼-希尔伯特问题时提出希尔伯特变换，而他关于离散希尔伯特变换的早期工作可追溯到他在哥根廷的讲课。
• Hermann Weyl在他的学位论文中发表了离散希尔伯特变换的结论。
• Schur改进了离散希尔伯特变换的结果，并将其扩展到了积分条件下。

而将Hilbert变换运用到信号处理中还得追溯到解析信号表达的建立。

Hint

“传统经典的信号研究方法主要概括为基于傅里叶变换的谱分析、基于概率分布的统计分析和其它随机信号表示方法，同时还有起源于很早的典型谱、相关和分布特征，而这些分析方法研究的一个基本考虑是将随机信号表达为两个独立函数的乘积”

早期关于包络和瞬时相位的研究都是基于笛卡尔坐标系x-y

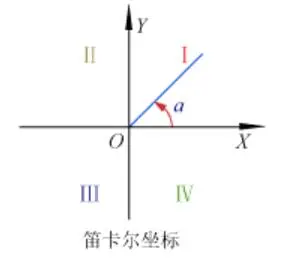
有关系：

$$\begin{array}{rl}{A}^2& =x^2+y^2\\ \phi & =\arctan \frac{y}{x}\end{array}$$

这样的表达被引入傅里叶序列中，$x_k=\sum a_k\cos {\phi }_k+b_k\sin {\phi }_k$, 其幅度和相位均可由上面笛卡尔坐标系中的两个关系得到，此时坐标$(x,y)$就是$(a_k,b_k)$。 用这种方法研究调制信号的包络和瞬时相位依赖于一个伟大的公式：

$$e^{i\phi }=\cos \phi +i\sin \phi$$

1946年， Gabor先生定义了复函数更一般化的欧拉公式

$$Y(t)=u(t)+iv(t)$$

这里的$v(t)$是$\mu (t)$的希尔伯特变换

1998年，Huang在现代希尔伯特变换研究领域做出了显著性工作 —— EMD、HHT，使得希尔伯特变换理论在现代信号分析中遍地开花

Analytical Signal

Mathematical description

The mathematical description of the Hilbert transform is to rotate the Fourier components in complex area.

$$H(\mu )(t)=\frac{1}{\pi }\text{p.v.}{\int }_{\infty }^{\infty }\frac{\mu (t)}{t-\tau }d\tau$$

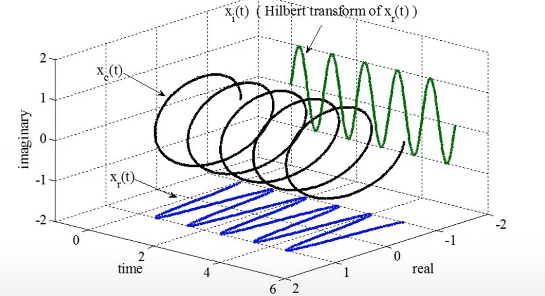
The Hilbert transform is given by the Cauchy principal value of the convolution with the function $1/(\pi t)$.

Geometrical meaning of HT

Reference

• Mathuranathan. “Extract Envelope, Phase Using Hilbert Transform: Demo.” GaussianWaves, 24 Apr. 2017, https://www.gaussianwaves.com/2017/04/extract-envelope-instantaneous-phase-frequency-hilbert-transform/.
• CFC: What Does the Hilbert Transform Do? (V9). www.youtube.com, https://www.youtube.com/watch?v=-CjnFEOopfw. Accessed 2 Jan. 2024.
• Extract Envelope and Fine Structure in Praat Using the Hilbert Transform. www.youtube.com, https://www.youtube.com/watch?v=qp1G3a2g8r0. Accessed 2 Jan. 2024.
• “希尔伯特变换与瞬时频率问题—连载（一）.” 知乎专栏, https://zhuanlan.zhihu.com/p/25213895. Accessed 2 Jan. 2024.
• The Hilbert Transform. www.youtube.com, https://www.youtube.com/watch?v=VyLU8hlhI-I. Accessed 3 Jan. 2024.